Disjoint Set 顧名思義,代表了一群兩兩交集為空的集合們,常用於處理依據某種關係將元素們「分類」的問題。我們的目標是去 maintain collection of disjoint sets $S_1,S_2,\cdots,S_k$,而每個 $S_i$ (每個 category) 以一個代表值 (representative) 去紀錄。
支援的 Operations
Make-Set(x): create a new set {$x$}Find(x): Find the leader of $x$'s setUnion(x,y): Union $x$'s set and $y$'s set
Linked List Implementation
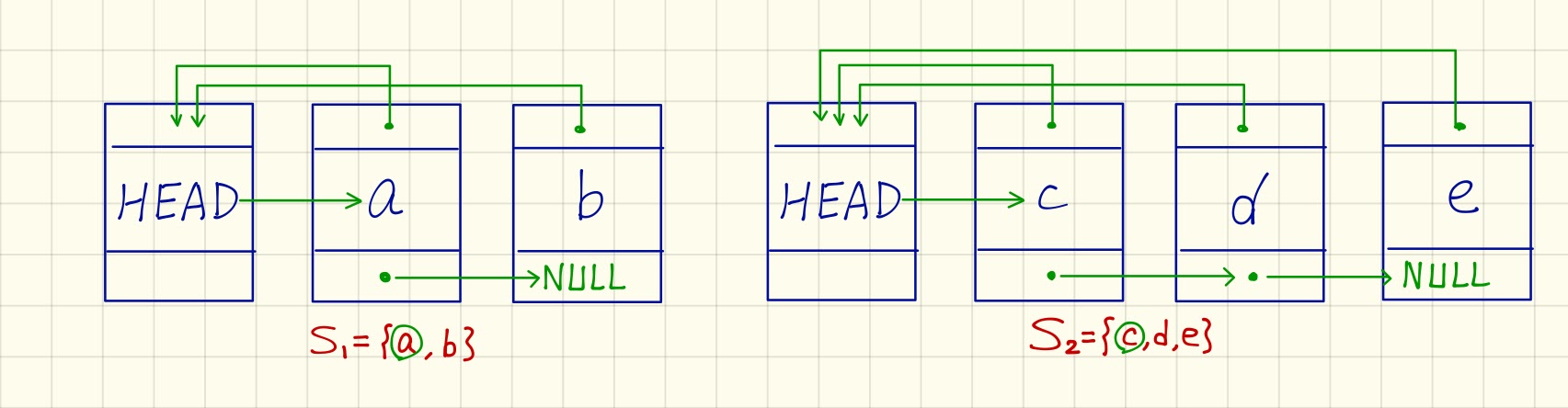
Make-Set(x): $\mathcal{O}(1)$ (createHEADand representative,也就是自己)Find(x): $\mathcal{O}(1)$ (back toHEAD,go to representative)Union(x,y): $\mathcal{O}(n)$ \
可以發現 Union 非常慢,要依序將 $S_2$ 中的元素依序指到 $S_1$ 的HEAD,同時將 $S_1$ 的最後一個元素之ptr指到原先 $S_2$ 中的第一個。(當然可以在HEAD中多存一個指向最後一個元素的ptr,但複雜度還是 $\mathcal{O}(m)$, where $m = |S_2|$)
雖說單看 Union 的複雜度似乎不太妙,但考慮一系列操作( Amortized Analysis ,也是一種常見的分析複雜度方式),同時利用上方提到的 trick ,在做 Union 時,都是去合併較小的那個集合,再來做分析。
Lemma
Starting from 0 sets
[
\text{operations} \left{ \begin{array}{ll}
n \quad \text{make-set} (, \therefore , n ; \text{elements})\
m \quad \text{find}\
\leq n-1 \quad \text{union}
\end{array} \right.
]
$\Rightarrow , \text{total cost}: \mathcal{O}(m+n \log n)$\
其中 $n\log n$ 顯然是來自 Union 的 cost,而細看操作會發現,這亦為 $\mathcal{\Theta}(\text{total number of times that element's top ptr changes})$,\
所以今天我們想證明,改動 ptr 的次數為 $\mathcal{O}(n \log n)$
Claim: If an element has changes ptr $k$ times, then it belongs to a set of
size $\geq 2^{k}$
用數學歸納法可以簡單證明,假設 $k=t$ 時成立,那在 $k=t+1$ 時,如果該 set 還需改動 ptr ,表示其跟 size 比他大的 set 做 Union ,之後 size $\geq 2^{t+1}$ , Claim 依然成立 ($k=0$ 時,只有 make-set, size 為 $1$ 亦成立)
而利用該 Claim ,又已知 size of any set $\leq n$,所以任何元素都只能改動 ptr $\leq \log n$ 次,所以 total ptr 改動次數 $\leq
n \log n \quad \square$
Forest Implementation
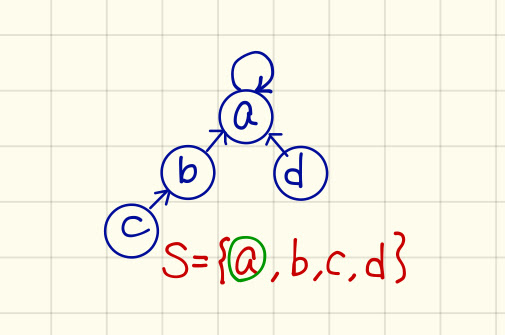
Make-Set(x): $\mathcal{O}(1)$ (建一顆只有一個節點的樹,root指向自己)Find(x): $\mathcal{O}(\text{height})$ (trace back to root)Union(x,y): $\mathcal{O}(\text{height})$ (單純改變被合併集 root 的指向為 $\mathcal{O}(1)$ ,但要先用Find找到 root)
稍微觀察一下發現,如果今天這個樹長的很傾斜(最爛的情況就是 skewed tree ,直接退化成 list),那麼 height 為 $\mathcal{O}(n)$ ,複雜度沒有任何改進。但若仿效前面 linked list 的想法,在做 Union 時,去合併比較小的 set ,則可以避免這個問題 (skewed tree會發生,就是每次都是拿一個 size 非 $1$ 的 set 去接在 size 為 $1$ 的 set 後面…)。
Theorem: The height of every tree $\leq \log n$
Claim: every tree with height $k$ has at least $2^{k-1}$ elements
- $k=1$, trivial
- 假設 $k=t$ 成立,考慮兩個高度同為 $t$ 的樹(如果不一樣的話,那合併後的樹高度為原先兩者中較高的那個,但元素個數又增加了,Claim 必成立),各自至少有 $2^{t-1}$ 個元素,合併後高度變為 $t+1$ ,元素個數 $\geq 2^{t-1} \times 2 = 2^{t}$, Claim 依然成立
所以當已知每顆樹至多 $n$ 個元素的情況下,我們可以證明其高度必 $\leq \log n \quad \square$
