Advanced Algorithm - Hash Table - Lec1

Intro & Analysis/ 2-Universal Family

Advanced Algorithm
Algorithm / Data Structure / NTU

Hashing 可以想成是一種 renaming 的方式，原先的名字 (key) 可能很長，但可能的組合並不完全隨機，且數量相對整個宇集少上不少，若我們要建立一個跟宇集一樣大的 Hash Table 並不符合成本(且大部份 slot 是空的)，所以想透由 Hashing 的方式，重新命名 key’ ，並依據 key’ 將資料放到 size 跟資料個數差不多的 Hash Table 中。

定義

Given each data with key in $U = \lbrace 0,1, \cdots, t-1 \rbrace$,

A Hash Table is capable of performing the following operations in $\mathcal{O}(1)$

  1. Insert(x)
  2. Delete(x)
  3. Search(x)

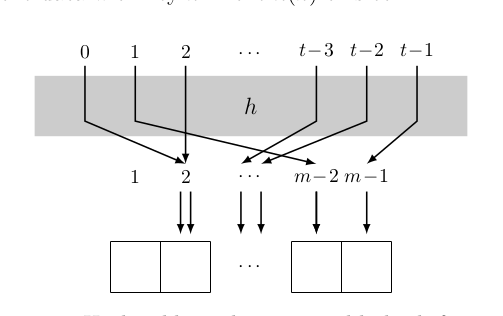
如同前面所述，$m \ll |U|$，所以hash function 必是 many-to-one mapping ，
也就是說，會有 collision 發生。而通常以下圖所示的 Chaining 來處理。

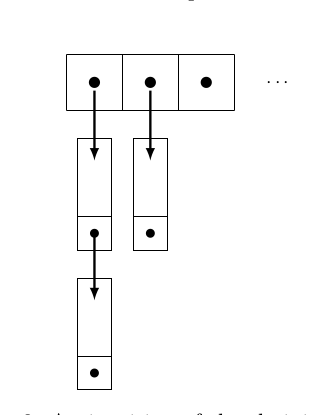
Hash Table 中所存的 element 為指向一個個 linked list 的 pointer。

在考慮 Chaining ，做複雜度的分析前，先假設我們有一 uniform hash function $h$，滿足以下性質

  • $\Pr[h(k) = x] = \frac{1}{m}, x \in \lbrace 0,1,\cdots, m-1 \rbrace$
  • $h(K_1),h(K_2),\cdots,h(K_n) \text{~are independent if} ~K_1,K_2,\cdots,K_n \text{~are independent}$

Average time complexity

[Theorem 1] Assume Uniform Hashing, $\mathbb{E}[op] = \mathcal{O}(\alpha)~~$ (Load Factor $~\alpha = \frac{ |\text{elements}|}{ |\text{slots}|} = \frac{n}{m}$)

可以觀察到，若 m = $\mathcal{O}(n)$，則 $\mathbb{E}[op] = \mathcal{O}(\alpha) = \mathcal{O}(1)$，
也就是說，只要 table size 跟元素個數是同個 order ，平均 來說，每個 operation 的 cost 還是 $\mathcal{O}(1)$。

Worst-case time complexity

現在我們想考慮在最差情況下的複雜度，
考慮一個類似的問題，將 $n$ 顆球 uniformly at random 地分到 $n$ 個 bin 中，
“the expected # of balls in the most loaded bin” 為何 ？

[Theorem 2] The max # of balls in any bin $\mathbf{\leq \frac{3\ln n}{\ln(\ln n)}}$ with probability $\mathbf{\geq 1 - \frac{1}{n}}$

$\underline{~Proof.} ~~~Let~k = \frac{3 \ln n}{\ln(\ln n)}~ \text{and}~ [S]^k \triangleq \lbrace A \subseteq S~ | ~|A| = k \rbrace$, $S$ is the set of balls

$$ \begin{aligned} \Pr[\text{Bin} \; i \; \text{has}\, \geq k \; \text{balls}] & \leq \sum_{A \in [S]^k} \Pr[\text{Every ball in}~ A~ \text{is in Bin}~ i~] ~~(\because \textbf{Union Bound}) \\\\ & = \binom{n}{k} (\frac{1}{n})^k ~~(\because \textbf{uniform hashing}) \\\\ & = \frac{n!}{k!(n-k)!} ~ \frac{1}{n^k} = \frac{1}{k!} ~ \frac{n!}{(n-k)!n^k} \\ & \leq \frac{1}{k!} ~~(~\because~ \prod_{i=0}^{k-1}n-i \leq n^k) \\ & \leq (\frac{e}{k})^k \, (\because \, \text{Stirling formula as follows})\\ & \leq \color{red}{\frac{1}{n^2}} \, (\color{red}{\text{when} \; k = \frac{3 \ln n}{\ln(\ln n)}} \; \text{and according to the following lemma}) \end{aligned} $$

[Theorem 3] Stirling's approx. formula

\[ k! = \sqrt{2 \pi k}~ (\frac{k}{e})^k (1 + \mathcal{O}( \frac{1}{k} )) \]

[Lemma] $\forall \epsilon \in \mathbb{R}^{+} ; \exists , n_0 , s.t. , \forall n > n_0 , \boxed{\ln (\ln n) \leq \epsilon \ln n}$

Let $k = \textcolor{red}{\frac{3 \ln n}{\ln (\ln n)}}$ $$ \begin{align} \therefore \ln (\frac{e}{k})^k &= k(1 - \ln k)\\ &= k[ \underset{\leq 0}{\underbrace{1 - \ln 3}} - \ln(\ln n) + \underset{\leq \frac{1}{3}\ln(\ln n)}{\underbrace{\ln(\ln(\ln n))}}] \\ &\leq k \cdot -\frac{2}{3} \ln(\ln n) = -2 \ln n \, \square\\ &\color{red}{\Rightarrow (\frac{e}{k})^k \leq \frac{1}{n^2}} \end{align} $$

Uniform Hashing?

看起來很合理，但當中我們做的一個假設其實是不合理的，也就是存在 uniform hashing function $h$ 這件事情，想完成這個條件只有以下兩種可能

  • input is random (impossible !!)
  • input is arbitary, but hash function is random

random hash function? 意思是說，我們希望有一組 hash function 的集合 $\mathcal{H}$，當每次要用到 hashing 時，便從中抽一個 $h: U \rightarrow \lbrace 0,1,\cdots,m-1 \rbrace$ 出來，可能結果很不好 ( collision 很嚴重， 可以想成總是可以構造出某些 input sequence ，讓特定的 $h$ 壞掉)，在這種情況可以再重新抽，平均而言 collision 發生的機率很小即可 (i.e 不用太常重抽)。

我們希望 $\mathcal{H}$ 有以下性質:
$\forall x_1,x_2,\cdots,x_n \in S,~S \subset U,~ \text{and}~ a_1,a_2,\cdots,a_n \in \lbrace 0,1,\cdots,m-1 \rbrace$

  • $\displaystyle \Pr_{h \in \mathcal{H}}[h(x_1) = a_1] = \frac{1}{m}$
  • $\displaystyle \Pr_{h \in \mathcal{H}}[h(x_1) = a_1 ~\land~ h(x_2) = a_2 ] = \frac{1}{m^2}$ **[Pairwise independence]**
  • $\displaystyle \Pr_{h \in \mathcal{H}}[h(x_1) = a_1 ~\land~ h(x_2) = a_2 ~\land~ \cdots ~\land~ h(x_n) = a_n ] = \frac{1}{m^k}$ **[k-wise independence]**

若想有 k-wise indep. 性質，則我們需要 $m^k$ 個 hash function $h$，需要 $\log |\mathcal{H}| = k \log m$ 個 bits 去記 $\mathcal{H}$ ，也就是說，若想要更好的隨機性， compute hashing 的成本就越高。

Let $L_x ~\text{be the length of linked list containing}~ x$

\[ I_y = \left\{ \begin{array}{ll} 1, ~\text{if}~ h(y) = h(x) \\ 0, ~\text{otherwise} \end{array} \right. \]
\[ \begin{aligned}

& \text{Then},~ L_x = 1 + \sum_{y \in S ;y \neq x} I_y \

& \text{We know~} \mathbb{E}[L_x] = 1 + \sum_{y \in S ;y \neq x} \mathbb{E}[I_y] = 1 + \frac{n-1}{m} \leq 1 + \alpha \end{aligned} ]

上例不需要用到 k-wise independence 這麼強的性質， pairwise independence 即符合我們的需要，
也因此 2-Universal Hash Family 應運而生。

2-Universal Hash Family

$\mathcal{H}$ is 2-Universal iff for and two elements $x,y \in U ~\land~ x \neq y$

\[ \Pr_{h \in \mathcal{H}}[h(x) = h(y)] \leq \frac{1}{m} \Leftrightarrow |\mathcal{H'}| \leq \frac{|\mathcal{H}|}{m}_ ~,~ \mathcal{H'} = \lbrace h ~|~ h(x) = h(y) \rbrace \]

Example:
Given $x=x_0 x_1 \cdots x_r~,y=y_0 y_1 \cdots y_r$, $a = < a_1,a_2,\cdots,a_r>,~a_i \in \lbrace 0,1,\cdots,m-1 \rbrace $

\[ h_a(x) = \sum_{i=0}^n a_i x_i \mod m \]
 
comments powered by Disqus